Aligning three circle intersection equally

Question

I am having trouble aligning my objects and I just rely on my eye to have them aligned. What I really want is to have the three "red" intersection to be equal in size

Please see image below.

I wanted the three circles to intersect at some point but I am not sure if they do intersect equally.

Here's what I did.

I align the top of the the two circle above and have them intersect at some point. And then align the bottom circle and have them intersect the two.

I just rely on my eye to see if they are aligned but I am thinking if illustrator has some tool to do this.

Answer 1

First create an equilateral triangle.

To do so use the Polygon Tool and bring the number of edges down to 3 using the keyboard arrows. Tap the down arrow to reduce the number of sides. Make sure you hold the Shift key down as you drag with the tool. This ensures the triangle is straight.

Then create your circle and align the center of the circle with one of the corners of the triangle. Enabling Smart Guides (View > Smart Guides) will help with alignment.

Duplicate the circle and place the second circle so it aligns with another corner of the triangle. Repeat this for the third circle.

Additional details:
You might want to have Align to pixel grid unchecked in the Transform Panel for more precision.

If you're unhappy with the size of your circles use the Scale Tool (s). Select one circle, press enter, specify a scale value. Then repeat for the other two circles one at a time.

Answer 2

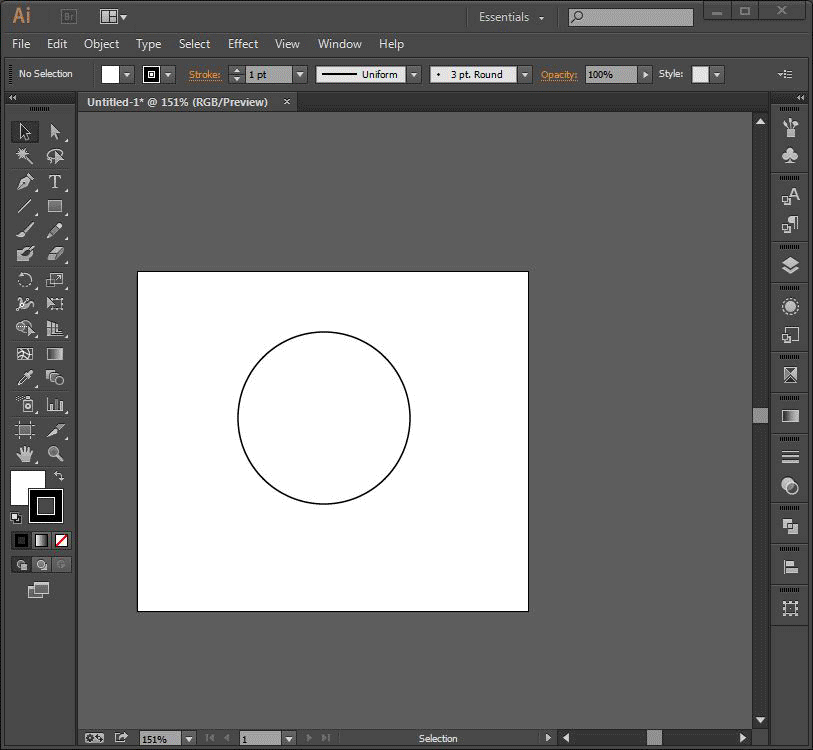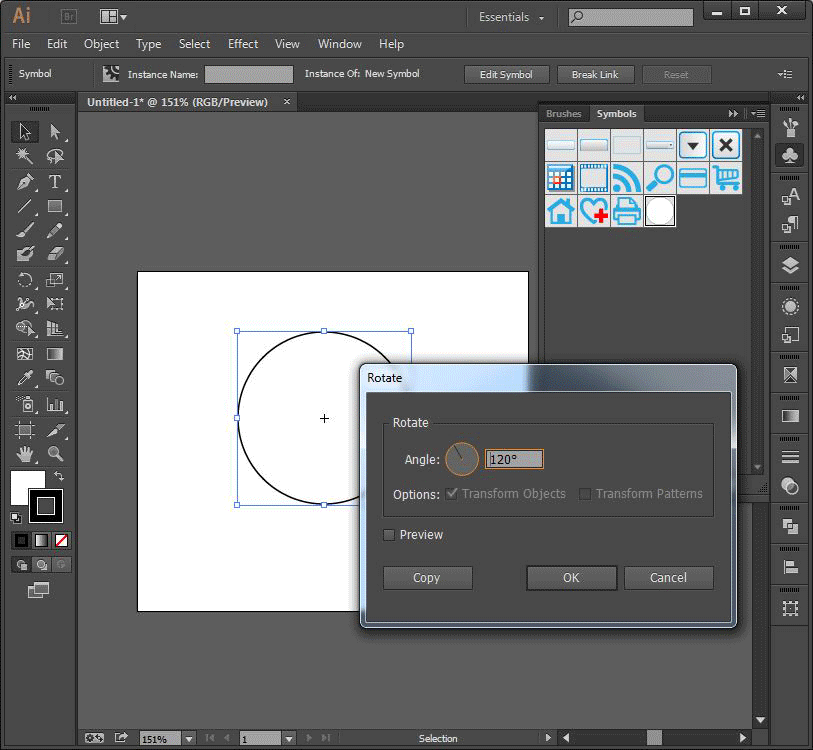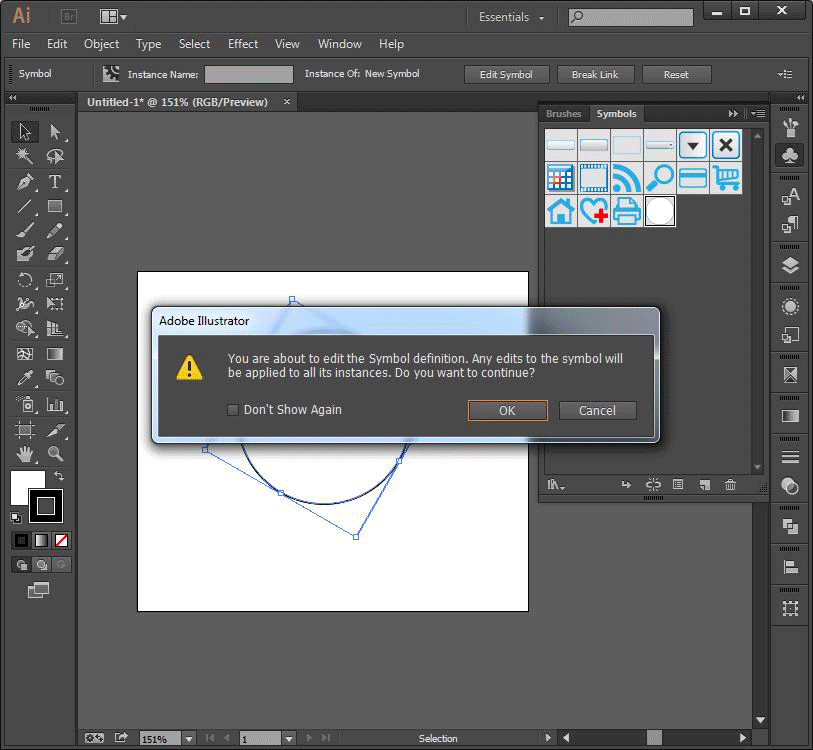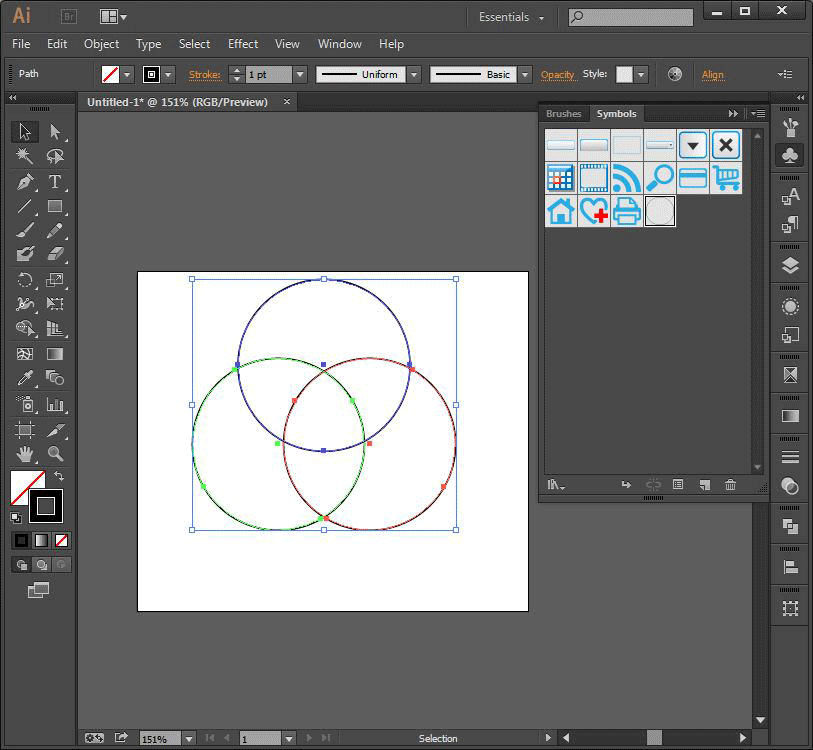
This funny answer for those who like math. It addresses the perfect shape where the center of one circle intersects with the circumference of 2 other ones, however from this explanation one can extrapolate how to achieve the equal distances for any given horizontal displacement.

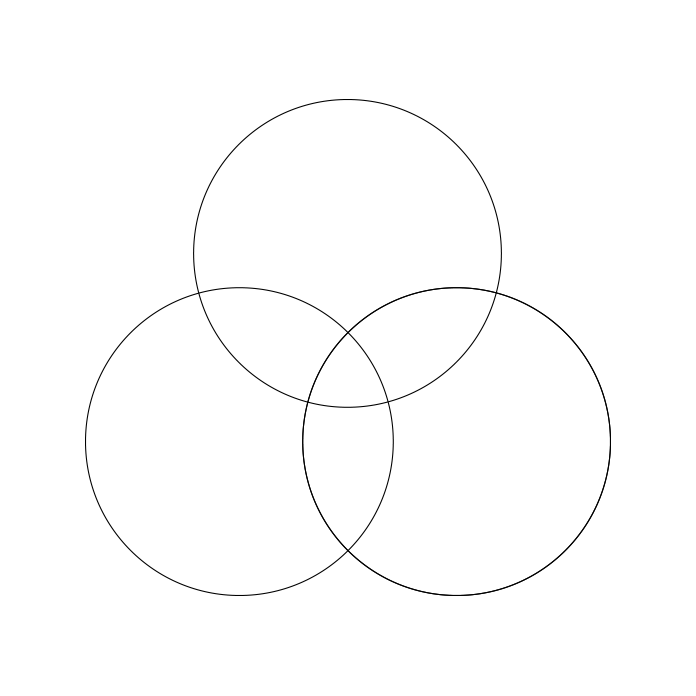
Let's look at this picture:

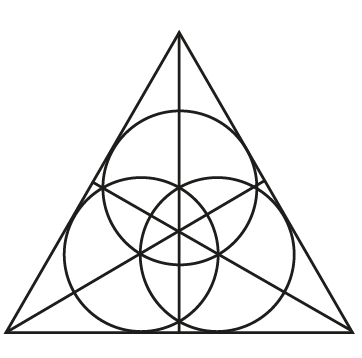
We placed two lower circles as described - at 1 distance of their radius.

We can clearly see that the horizontal displacement of the upper circle will always be at 1/2 distance between the lower ones, i.e. 1/2 of the radius.

Second, the centers of the circles are at the radius distance.

Now, we have (I know you feel what I want to do):

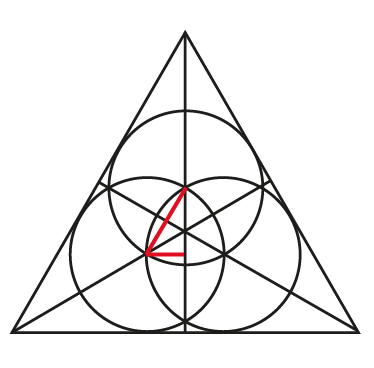
So, we have a hypotenuse (r in our case), we have a small cathetus (r/2)... and what we left to do is... to calculate the long cathetus, which is naturally the consequence of Pythagorean theorem.

Thus, long cathetus = √ (r²-0.5r²).

So, we create one circle and horizontally displace its copy to 1 radius distance.

Next, we displace another copy horizontally to 1/2 of the radius, and vertically accordingly to the calculated value of long cathetus - with minus sign before it:

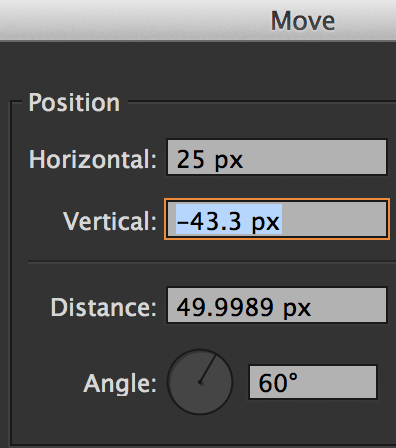
And that what we get:

Another case for arbitrary horizontal displacement -

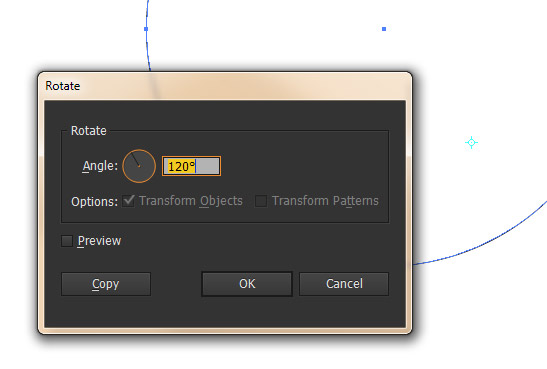
Sad to say, if we could displace the circle by the angle and distance of displacement via transform operation we could achieve perfect results, however, Illustrator convert the distance of displacement incorrectly to vertical displacement after the angle is inserted.

Answer 3

To have all three circles intersect equally make sure to align the center points of all the circles on the edges of the other 2 circles.

There is no specific tool in Illustrator that will do this for you however you can use guides and the visual cues when the shapes intersect.

Answer 4

Provided you don't really care about the resulting shape not being perfectly horizontally aligned, you can use this quick method:

• Draw a circle;
• Select it;
• Grab the Rotate tool (R);
• L-Alt+click somewhere inside your circle;
• In the dialog box that pops up, choose 120° for your angle and click Copy;

• Choose Object > Transform > Transform Again (Ctrl/Option+D) to repeat once.

Answer 5

Grab the three circles and using the Align panel there are some Distribute Objects options, you want one thats called Distribute Horizontal Centers (5th one from the right looks like some centered blocks on sticks), that puts them all nicely spaced.

Then we want to check out the Align Objects part of that same Align Panel. Hit the Vertical Align Top, that gets them neat in a row.

Now grab the middle one and pull it down, odds are it will 'click' into place if you have some of the Snap To settings on. If you need to put them on, have a look in the 'View' menu up top OR Cmd+U turns on Smart Guides and Alt+Cmd+' will pop on the snap to.

Hopefully this should get you where you want to be!

Answer 6

Another method, just for fun. The basic idea is, the art you want to achieve is made up a circle translated away from its center and then roated 120 degrees twice. I use a symbol to encapsulate that basic shape (the symbol translated and rotated).

1. Draw a circle
2. Turn it into a symbol. With the symbol window open (Window->Symbol or Ctrl+Shift+F11), click on the top right corner, select New Symbol and press OK.
3. Select from the menu Object->Transform->Rotate, enter 120 degrees as the Angle and click on Copy, not on OK. A copy of the symbol circle is created on top of the original one, but rotated 120 degrees.
4. Now with the new circle selected (the top most), repeat the previous step again to create a 3rd copy of the symbol, again rotated 120 degrees (240 degrees from the original one).
5. Now that we have 3 copies of the circle, rotated, we need to translate them. As opposed to doing it for each one of the copies, we will do it to the symbol definition, so it gets replicated in every symbol instance. Double click on the top most circle symbol to enter the symbol edition mode. If the circle you draw has no fill (transparent fill) then you will have to double click on the outline of the circle. If a window appears alerting you that you are about to edit the symbol, click OK.
6. Move the circle upwards using the method of your choice. For example, using the selection tool (V, black arrow) and keeping shift pressed (to restrict movement to straight angles) drag the circle upwards. Notice that the center of the circle is not aligned anymore with the registration center of the symbol (the little cross in the middle of the symbol).
7. Click anywhere outside the symbol art to exit the symbol editing mode. The other 2 symbols mimic the transformation (they move away from the registration).
8. Select the 3 symbols and break the link to the symbol, if you wish (using the symbol window, click on the top left corner and select Break link to symbol) so they are not symbols anymore but just plain circles.

Here is a rough animation of the process.