0

I'm trying to figure out on how can I make symmetric Bezier curves.

Symmetric here means something different than InkScape's interpretation:

Consider this path segment:

enter image description here

I want to make it symmetric to each other like this:

enter image description here

So I'm looking for the key-combination or technique to grab only one side of a path, and adjust both ends symmetrically, but horizontally mirrored.

How can I achieve this?

(If I don't want to create a half path, and then mirror it, then rotate it.)

Improve this question
9
  • 1
    Snap to grid make handles symmetric. See i.sstatic.net/yAOyy.gif
    – joojaa
    Commented Apr 6, 2022 at 20:34
  • That only works if the line is either horizontal or vertical (based on my understanding) am I missing something here?
    – Daniel
    Commented Apr 7, 2022 at 6:24
  • Well no, just as long as your tangents can snap to your grid. Just make the grid suitably small. For your usecase the grid is almost certainly good enough. The grid just has the same function as rounding numbers just choose a rounding thats appropriate and thats that. But in reality you can rotate the grid to fit your objective. So there is never a situation where its not aligned to the bezier.
    – joojaa
    Commented Apr 7, 2022 at 7:21
  • Aham, and there is no way to grab only one handle and make the other handle mirror the distance and angle?
    – Daniel
    Commented Apr 7, 2022 at 8:47
  • @Daniel There is. You can use a Smooth node for that, but it won't necessarily make the curve geometrically symmetric. You would still need a grid to align it to.
    – Billy Kerr
    Commented Apr 7, 2022 at 9:07

1 Answer 1

Reset to default
2

I must admit that I'm not sure what your symmetry concept means. But your battered thick curve may present it perfectly for you because you can filter out the bumps and see the essence. It probably is so clear for you that you do not see any reason to formulate it as a math relation.

Unfortunately I do not have the same inner sight. But I guess: You want a circular arc. It surely is symmetric in relative some axis (see NOTE1)

Actually you should decide a third condition to make only one arc valid. If only 2 points are declared, infinitely many arcs will fit.

An example:

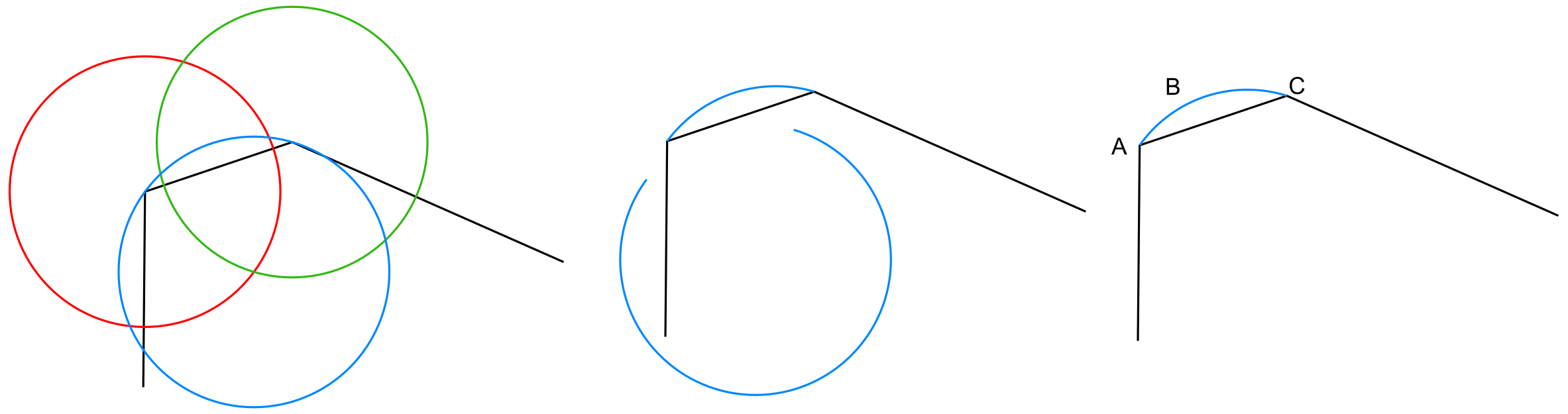
enter image description here

In the left the red circle was drawn first. It was duplicated. The duplicate was colored to green and moved to the other wanted point. The blue circle is also a duplicate of the red one, but moved to the crossing of red and green circles.

The circles snap exactly if you have node, path and center snappings ON.

In the middle the blue circle is split at the corners with the node tool and the unwanted part is moved away.

In the right an arc is drawn directly with the Bezier tool. It draws arcs in Spiro mode. Only click at 3 points (A, B, C) of the arc. The intermediate point B is arbitary as long as there's no 3rd condition declared.

You can move the intermediate point B afterwards with the node tool for visually right appearance. If you want to continue drawing from the ends of the Spiro path by drawing usual Bezier paths you must at first convert the Spiro path to an ordinary path by applying Path > Object to Path.

NOTE1: the symmetry axis is the middle normal of line segment AC in the rightmost image

Improve this answer
4
  • This is nice, thx. And yes, I know there are infinite number of symmetric circles that are touching both A and B. I do not know the spiro but will check. A B and C are also meaning the click order? I.e. A is first point of Spiro, B is Spiro's height, and C is the endpoint?
    – Daniel
    Commented Apr 7, 2022 at 12:34
  • The clicking order is ABC. Stop drawing by right clicking. The result is the only possible circular arc which contains points A, B and C. B doesn't present any height. Draw at first the mid normal of the straight line segment AC and click B on it. Then B can be seen as a height. Draw a line AC and rotate it 90 degrees to make it the mid normal.
    – user82991
    Commented Apr 7, 2022 at 12:38
  • I see. So this will always be symmetric between A and C (as its circular arc)?
    – Daniel
    Commented Apr 7, 2022 at 12:42
  • 1
    The arc is symmetric when the mid normal of straight line AC is considered as mirror.
    – user82991
    Commented Apr 7, 2022 at 12:44

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.